Others

TH-pentagon

There exists a convex pentagon that can be considered as a unique convex pentagon obtained from a trisected heptiamond. The convex pentagon is referred to as a TH-pentagon. I found novel properties of the TH-pentagon and many new convex pentagon tilings.

 

Monotile that admits only non-periodic tiling

If all the tiles in a tiling are of the same size and shape, then the tiling is monohedral, and the tile is called a monotile. Note that, in monohedral tiling, it admits the use of reflected tiles.

Aperiodic set of prototiles (Wiki)
"A set of prototiles is aperiodic if copies of the prototiles can be assembled to create tilings, such that all possible tessellation patterns are non-periodic. The aperiodicity referred to is a property of the particular set of prototiles; the various resulting tilings themselves are just non-periodic."

The following paper, in which an Aperiodic monotile was found, was published on the arXiv on March 20, 2023.

An aperiodic monotile　https://arxiv.org/abs/2303.10798

David Smith, Joseph Samuel Myers, Craig S. Kaplan, and Chaim Goodman-Strauss

I noticed certain properties with this monotile and summarized them in

'Belts that have tile pavement pattern with periodicity by aperiodic monotile "hat"',

'Properties of the Fibonacci sequence and two-fold rotational symmetry in clusters formed by aperiodic monotile "hat',　

'Relationship between aperiodic monotiles of "hat" and "turtle" and Type 5 tiling of convex pentagonal tiles' ,

'Presentation of the results of the conversion of the non-periodic tiling with Tile(1,1) to a tiling with three types of pentagons',

and

'Supplement of the paper "Converting non-periodic tiling with Tile(1, 1) to tilings with three types of pentagons"'.

Introduction of models (in Japanese)

Type 15の凸五角形タイルの模型

積み木インテリアギャラリーから，Type 15の凸五角形タイルの模型をいただきました．

 

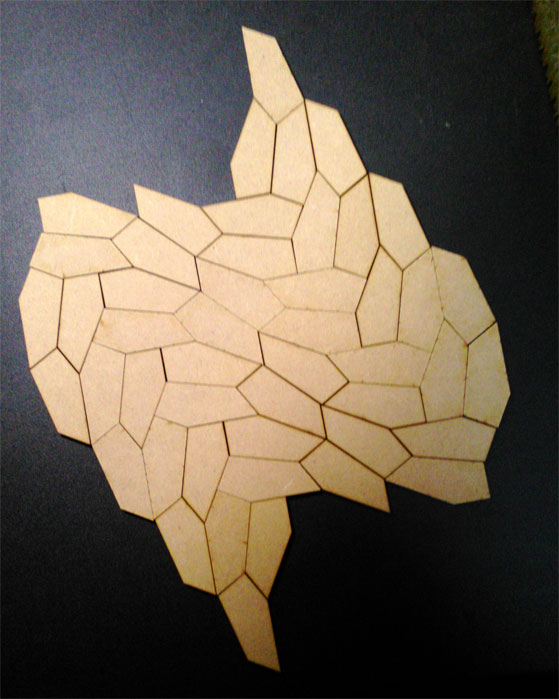
72°の内角を2つ備えた4等辺凸五角形を使ってタイリングを作ってみよう！

木工職人の中川宏さんと知り合う機会があり，中川さんに下の写真のような凸五角形タイルの木製模型を作って頂いた．この模型を使って実際にタイリングを作ることを経験してもらいたいと思い，第71回の形の科学シンポジウムにて展示を行った．